How to get countably many partials of any tone you like in a very quick way
This program is dedicated to all friends of spectral music as well as composers and music theorists who have spent hours of their precious time writing down harmonic series of a given fundamental, calculate all the partials meticulously using a pocket calculator and comparing two or more overtone series on a piece of sheet music. Here’s the good news: There’s no need to make things more complicated than they should be. Let’s get all the numeracy done by using the computer. Remember the meaning of the word «computer»—computing things belongs to its core skills. And it keeps computing things flawlessly.
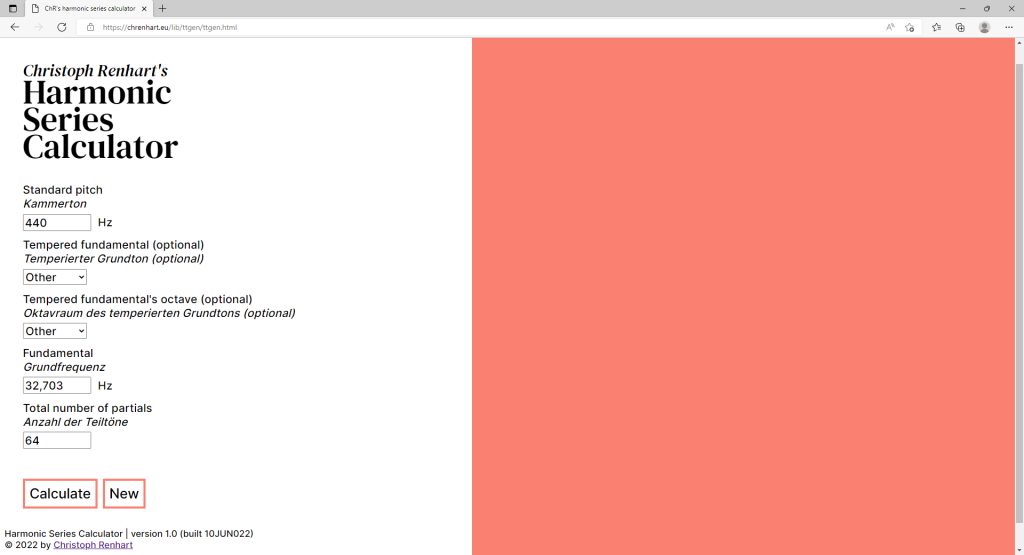
What can be done with this program? Suppose we would like to get the first 32 partials of the tone E2. Select E as tempered fundamental and 2 from the list of octaves (remember: C1 is the lowest C on the piano). Next click on Calculate and find the results below. In the table of results you will see the exact frequecy of each partial as well as its pitch (e.g. the 7th partial of a tone with a fundamental frequency of 32.703 Hz (C1) is 228.921 Hz which is A#3 minus 31 Cents).
Naturally all the frequencies depend significantly on the standard pitch. So we might want to compare the 17th partial on A2 using 440 Hz as a concert pitch with the 17th partial of A2 using 443 Hz as standard pitch. This can also done easily with the program. Let’s do the 440 Hz first:
- Enter 440 Hz as standard pitch
- Select A as tempered fundamental
- Select 2 as octave
- Click Calculate
Next, let’s run the program again with a different standard pitch:
- Enter 443 Hz as standard pitch
- Select A as tempered fundamental
- Select 2 as octave
- Click Calculate
Finally, compare the two results:
Fundamental A2 — 440 Hz standard pitch:
| 1 | 110.000 | A2 plus 0 Cents |
| 2 | 220.000 | A3 plus 0 Cents |
| 3 | 330.000 | E4 plus 2 Cents |
| 4 | 440.000 | A4 plus 0 Cents |
| 5 | 550.000 | C#5 minus 14 Cents |
| 6 | 660.000 | E5 plus 2 Cents |
| 7 | 770.000 | G5 minus 31 Cents |
| 8 | 880.000 | A5 plus 0 Cents |
| 9 | 990.000 | B5 plus 4 Cents |
| 10 | 1100.000 | C#6 minus 14 Cents |
| 11 | 1210.000 | D#6 minus 49 Cents |
| 12 | 1320.000 | E6 plus 2 Cents |
| 13 | 1430.000 | F6 plus 41 Cents |
| 14 | 1540.000 | G6 minus 31 Cents |
| 15 | 1650.000 | G#6 minus 12 Cents |
| 16 | 1760.000 | A6 plus 0 Cents |
| 17 | 1870.000 | A#6 plus 5 Cents |
Fundamental A2 — 443 Hz standard pitch:
| 1 | 110.750 | A2 plus 0 Cents |
| 2 | 221.500 | A3 plus 0 Cents |
| 3 | 332.250 | E4 plus 2 Cents |
| 4 | 443.000 | A4 plus 0 Cents |
| 5 | 553.750 | C#5 minus 14 Cents |
| 6 | 664.500 | E5 plus 2 Cents |
| 7 | 775.250 | G5 minus 31 Cents |
| 8 | 886.000 | A5 plus 0 Cents |
| 9 | 996.750 | B5 plus 4 Cents |
| 10 | 1107.500 | C#6 minus 14 Cents |
| 11 | 1218.250 | D#6 minus 49 Cents |
| 12 | 1329.000 | E6 plus 2 Cents |
| 13 | 1439.750 | F6 plus 41 Cents |
| 14 | 1550.500 | G6 minus 31 Cents |
| 15 | 1661.250 | G#6 minus 12 Cents |
| 16 | 1772.000 | A6 plus 0 Cents |
| 17 | 1882.750 | A#6 plus 5 Cents |
As you can see, the frequencies differ from each other. With 440 Hz as concert pitch the 17th partial of A2 is 1870 Hz (A#6 + 5 Ct.), whereas using a concert pitch of 443 Hz the 17th partial of A2 is 1882.75 Hz (also A#6 + 5 Ct. of course).
Moreover, you can also choose a non-tempered fundamental frequency in relation to any concert pitch you like. Let’s say, for instance, our concert pitch is 443 Hz and our fundamental is 60 Hz. As the fundamental is (possibly) not equal to a tempered tone, we select Other from the list of pitches and also Other from the list of octaves (it was somehow difficult to find an appropriate name for the input field and perhaps I’m gonna rename it as Other is admittingly not ideal here). Finally enter 60 in the field Fundamental / Grundfrequenz. Choose any amount of partials and click Calculate. Let’s have a look at the first 32 partials:
| Number | Frequency | Tone |
|---|---|---|
| 1 | 60.000 | A#1 plus 39 Cents |
| 2 | 120.000 | A#2 plus 39 Cents |
| 3 | 180.000 | F3 plus 41 Cents |
| 4 | 240.000 | A#3 plus 39 Cents |
| 5 | 300.000 | D4 plus 25 Cents |
| 6 | 360.000 | F4 plus 41 Cents |
| 7 | 420.000 | G#4 plus 8 Cents |
| 8 | 480.000 | A#4 plus 39 Cents |
| 9 | 540.000 | C5 plus 43 Cents |
| 10 | 600.000 | D5 plus 25 Cents |
| 11 | 660.000 | E5 minus 10 Cents |
| 12 | 720.000 | F5 plus 41 Cents |
| 13 | 780.000 | G5 minus 21 Cents |
| 14 | 840.000 | G#5 plus 8 Cents |
| 15 | 900.000 | A5 plus 27 Cents |
| 16 | 960.000 | A#5 plus 39 Cents |
| 17 | 1020.000 | B5 plus 44 Cents |
| 18 | 1080.000 | C6 plus 43 Cents |
| 19 | 1140.000 | C#6 plus 36 Cents |
| 20 | 1200.000 | D6 plus 25 Cents |
| 21 | 1260.000 | D#6 plus 10 Cents |
| 22 | 1320.000 | E6 minus 10 Cents |
| 23 | 1380.000 | F6 minus 33 Cents |
| 24 | 1440.000 | F6 plus 41 Cents |
| 25 | 1500.000 | F#6 plus 12 Cents |
| 26 | 1560.000 | G6 minus 21 Cents |
| 27 | 1620.000 | G6 plus 45 Cents |
| 28 | 1680.000 | G#6 plus 8 Cents |
| 29 | 1740.000 | A6 minus 32 Cents |
| 30 | 1800.000 | A6 plus 27 Cents |
| 31 | 1860.000 | A#6 minus 16 Cents |
| 32 | 1920.000 | A#6 plus 39 Cents |
That’s it. Try it out and feel free to use it whenever you need to work with harmonic series.
Link to the calculator:
https://chrenhart.eu/lib/ttgen/ttgen.html
Special thanks to Daniel Mayer for checking the results with a SuperCollider patch and for some great advise to make the program’s interface more user-friendly!